Read About Long Division (Standard Algorithm for Division)

Divide a 3-Digit Number by a 1-Digit Number A batch of elephant toothpaste needs 936 milligrams of hydrogen peroxide divided equally among 4 containers. Divide 936 ÷ 4 to find how many milligrams go into each container. The standard algorithm for long division is a series of steps repeated in this order: divide, multiply, subtract, bring down. With the standard algorithm, we solve division problems one place value at a time. Start with the 9 in 938. Multiply: What multiple of 4 is closest to (but less than) 9? 4 × 2 = 8. Record a 2 in the hundreds place above the 9. Subtract: 9 -8 =1. Bring down: the next digit in 938 is 3. Write it next to the 1 to make 13. Repeat all the steps. 4 × 3 = 12, which is closest to 13. Write a 3 in the tens place above the line. Subtract 13 – 12 to get 1. Bring down the last digit, 6 to make 16. Repeat the steps again. 4 × 4 = 16. Write a 4 above the ones place. 16 – 16 = 0. There are no digits left to bring down, so you are done! The number above the line is 234 and the last subtraction resulted in 0. 936 ÷ 4 = 234. Try this one yourself: What is 875 ÷ 7?

Divide a 4-Digit Number by a 1-Digit Number You want to complete a trip of 1,421 miles in 6 days. Divide 1,421 ÷ 6 to find how many miles you must drive each day. To divide using the standard algorithm, write 1,421 under the division symbol and 6 to the left. Use the steps multiply, subtract, and bring down to find the quotient. What multiple of 6 is closest to 14? 6 × 2 = 12. Write a 2 in the hundreds position. Subtract: 14 – 12 = 2. Bring down the next digit, 2, to make 22. What multiple of 6 is closest to 22? 6 × 3 = 18. Write 3 in the tens position. Subtract: 22 – 18 = 4. Bring down the next digit, 1, to make 41. What multiple of 6 is closest to 41? 6 × 6 = 36. Write 6 above the ones position. Subtract: 41 – 36 = 5. There are no more digits to bring down. 5 is less than 6 so 5 cannot be evenly divided into 6 groups. 1,421 ÷ 6 = 236 with a remainder of 5. You need to drive a little more than 236 miles each day. Try this one yourself: Divide 2,576 ÷ 8.

Divide a 3-Digit Number by a 2-Digit number You want to divide a batch of 384 cookies among 12 middle school teachers. Solve 384 ÷ 12 using the standard algorithm for long division. The same process works no matter how many digits are in the divisor or dividend. Start by asking what multiple of 12 is less than or equal to 38? 12 × 3 = 36, so write 3 in the tens place and subtract to find the remainder: 38 – 36 = 2. Bring down the 4 to make 24. What multiple of 12 is closest to 24? 12 × 2 = 24, so write 2 in the ones position and subtract to find the remainder 24 – 24 = 0.There are no more digits to bring down. 384 ÷ 12 = 32. You can give each teacher 32 cookies. Yum! Try this yourself: What is 224 ÷ 32?

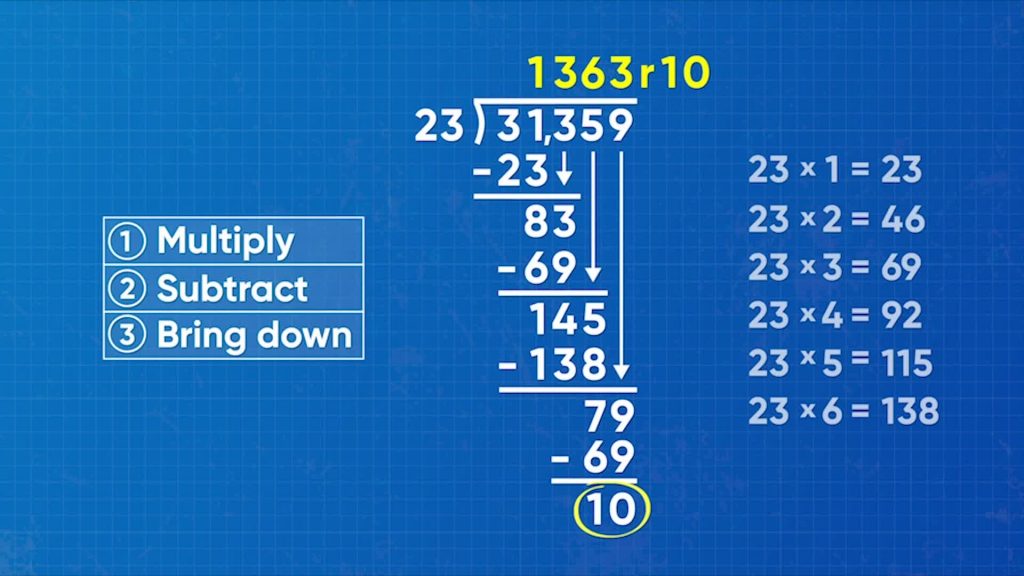
Divide a Large Multi-Digit Number by a Multi-Digit Number It takes 23 bees to pollinate one apple tree. If we have a total of 31,359 bees, how many apple trees can they pollinate? Solve 31,359 ÷ 23 by using the standard algorithm for long division. Consider the first two digits of 31,359. What multiple of 23 is closest to 31? 23 × 1 = 23, so write a 1 in the thousands place and subtract to find the difference: 31 – 23 = 8. Bring down the 3 to make 83. 23 × 3 = 69, so write 3 in the hundreds place and subtract to find the difference: 83 – 69 = 14. Bring down the 5 to make 145. 23×6=138, so write 6 in the tens place and subtract 145-138 =7. Bring down the 9 to make 79. 23×3=69, so write 3 in the ones place and subtract 79-69=10. There are no more digits to bring down and 10 cannot be divided into 23 equal groups. 31,359 ÷ 23 = 1,363 with a remainder of 10. The bees can pollinate 1,363 apple trees. Try this yourself: What is 53,922 ÷ 43?