Read About Intro to Finding Area

Somersaults Area is measured in square units. Let’s say you want to make a floor mat for a tumbling class. You put 5 rows that each have 2 square pads. You can count the number of squares to find the area you can tumble on and find that the area is 10 square units. Try this one yourself: You lay 24 square pads in a rectangular mat for a wrestling class. Then, you pick up 4 of the pads. What is the area of the new mat?

Defenders of the Area Different shapes can have the same area, as long as the same number of square units are needed to fill the space. You can also add or remove square units for a larger or smaller area. Let’s say you have a cape that is made of 27 squares of fabric of the same size. It is 9 squares long and 3 squares wide. You remove 6 squares from the bottom of the cape and attach them to a side to use as a hood. The area of your cape is still 27 square units. Try this one yourself: You use 12 square units of fabric to make a cape that is 3 squares long and 4 squares wide. Your cape is not long enough, so you add 9 more squares at the bottom. What is the area of your cape now?

Poster Array The area of a rectangle or square can be thought of as an array, with rows and columns of square units. To find the number of square units in the shape, you can multiply the number of columns by the number of rows. This is the same as multiplying the length by the width. Let’s say you have square posters that you are using to cover a wall. The wall fits 5 posters high and 5 posters wide. You can multiply 5 times 5 to find that the total area of the wall is 25 square posters. Try this one yourself: One-foot square posters are used to cover a wall. The wall is 6 posters high and 4 posters wide. What is the area covered by the posters?

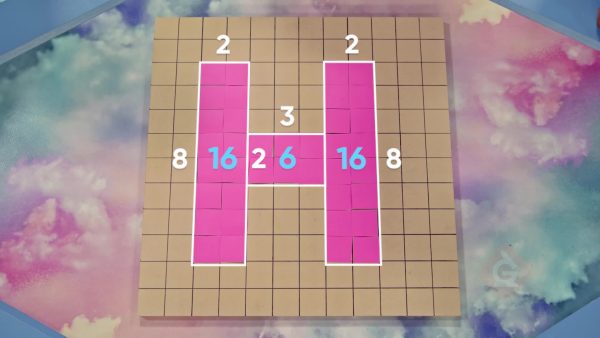
Pixel Art Shapes are not always perfect squares or rectangles. Sometimes you can break shapes apart into squares and rectangles to find their area. Let’s say you have a T-shaped figure that can be broken into a 2 × 2 square and a 6 × 2 rectangle. You can multiply to find that the area of the square is 4 square units and the area of the rectangle is 12 square units. Then, you can add the two areas to find that the area of the full shape is 4 + 12 = 16 square units. Try this one yourself: You have a U-shaped figure that can be broken into 2 rectangles that are 6 units long and 3 units wide and one square with a side length of 3 units. What is the area of the figure?